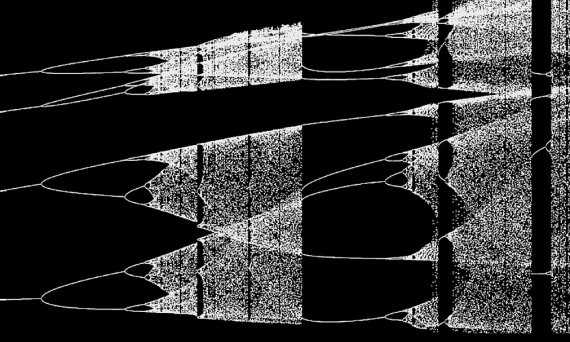
We extend the Keynesian Beauty Contest game to a second dimension. In this new, «planar beauty contest» game, groups of subjects are asked to simultaneously form guesses about two variables, X and Y, each in a closed interval [0, 100]. Average guesses matter for the realizations of the two target values for X and Y that subjects are incentivized to achieve, i.e., the system of equations is coupled. Such coupled systems represent a workhorse, reduced-form framework for many models in macroeconomics and other fields. Our planar beauty contest game provides a simple framework for understanding whether individuals can learn the steady state equilibria of such models and stands in contrast to «learning-to-forecast» experimental designs. Our main focus is on whether the eigenvalues of the induced twodimensional system matter for whether or not subjects converge to the steady state solution of the two-dimensional system, as well as the learning process by which such convergence (or divergence) takes place. We find that groups of 10 subjects with full knowledge of the planar model's two equations are able to learn the steady state of such systems when the steady state exhibits the sink property (two eigenvalues less than one in absolute value) or the saddlepath property with negative feedback, (one eigenvalue less than unity while the other eigenvalue is greater than unity and negative). However, if the steady state exhibits the source property (two eigenvalues greater than one in absolute value) or the saddlepath property with positive feedback, (one eigenvalue is less than unity while the other eigenvalue is greater than unity and positive), then subjects are unable to learn the steady state solution of the system, and instead get stuck at boundary values of the admissible solution space. We find that several different learning approaches can explain these phenomena, with a simple adaptive expectations model providing the best fit across all four of our experimental treatments.